今天在网上看到一个神奇的东西：把从 1/19 到 18/19 的所有分数展开成小数，得到一个 18 × 18 的数字方阵。这个数字方阵有什么特别的地方呢？
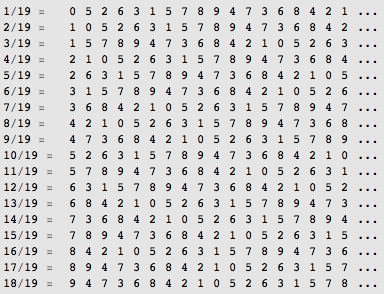
答案是，它是一个幻方——每一行、每一列和两条对角线上的数字之和都是 81 （注：严格意义上说它不算幻方，因为有相同数字）。
当然，相信大家像我一样，看到上图之后第一件事情就是验证把 19 换成 7 能否得到一个 6 阶幻方。嗯，我已经试过了，不能——对角线上的数不等于循环节内的数字之和。嗯，我已经把17、23、29之类的都试过了，都不能形成幻方。
19 这个数字有什么特别的地方？这真的只是一个巧合吗？
沙发！
沙发。新年好。
真早……
而且每一行每一列都有 1个0,两个2 3 4 5 6 7 8 9
发现 第一行通过 循环左移1位 等于 第二行
总之下一行的结果可以通过循环移X位上一行得到
还有383。另外17和23等某些素数只是对角线的和不一样
首页留名。
新年快乐~
二进制的1/3和2/3也符合要求.
或许k进制的 1/(2k-1)系列都符合要求.
楼上说的似乎有点道理
哎呀我错了…
1/3是 01
2/3是 10
对角线的和一个是0一个是2,差远了…
如果不考虑对角线的话,3进制的 1/5,2/5,3/5,4/5是很不错的 :)
0121
1012
1210
2101
数列百科全书里列举了这个属性的质数http://oeis.org/A072359
不过好像没有什么深入研究
怎么看都是巧合……
很神奇
围棋现在的棋盘是19X19，这个只是巧合吗：）
19的循环节长度为18，所以写一个18*18的矩阵满足这个性质
同样的，对于7，你写一个6*6的矩阵也满足这个性质，因为7的循环节长度是6
那么一个数字n的循环节长度为n-1，什么数才满足这个条件呢。
在10进制加法下，如果一个素数的原跟是10，那么它就满足这个性质
19，7的原跟都是10，所以在10进制下，可以造出上述矩阵。。。
哦，忘了对角线，无视我刚才说的吧
其实楼上都说的差不多了，因为是循环小数，横，竖的和一定都一样，只是对角线问题，因此我的观点同14L的仁兄相同：怎么看都是巧合……
我是来长见识滴。
9 進位表示 1/17,2/17,….16/17 也有幻方特性
0 4 6 7 8 4 2 1 0 4 6 7 8 4 2 1
1 0 4 6 7 8 4 2 1 0 4 6 7 8 4 2
1 5 2 5 7 3 6 3 1 5 2 5 7 3 6 3
2 1 0 4 6 7 8 4 2 1 0 4 6 7 8 4
2 5 7 3 6 3 1 5 2 5 7 3 6 3 1 5
3 1 5 2 5 7 3 6 3 1 5 2 5 7 3 6
3 6 3 1 5 2 5 7 3 6 3 1 5 2 5 7
4 2 1 0 4 6 7 8 4 2 1 0 4 6 7 8
4 6 7 8 4 2 1 0 4 6 7 8 4 2 1 0
5 2 5 7 3 6 3 1 5 2 5 7 3 6 3 1
5 7 3 6 3 1 5 2 5 7 3 6 3 1 5 2
6 3 1 5 2 5 7 3 6 3 1 5 2 5 7 3
6 7 8 4 2 1 0 4 6 7 8 4 2 1 0 4
7 3 6 3 1 5 2 5 7 3 6 3 1 5 2 5
7 8 4 2 1 0 4 6 7 8 4 2 1 0 4 6
8 4 2 1 0 4 6 7 8 4 2 1 0 4 6 7
各方向和為 64
每一个数字都有自己一定的独特吧~
巧合吧…
19岁的时候阴阳历生日同一天。巧合？
19, 383, 32327, 34061, 45341, 61967, 65699, 117541, 158771, 405817, 444287, 456503, 695389, 724781, 1102567, 1177859, 1498139, 2336989, 2695337, 3036857, 3249419, 3512749, 3571429, 4427299, 5141051, 7033823, 8324411, 9932179
See for reference: A072359 in http://oeis.org/
太神奇了！这究竟是怎么发现的？好奇死了
因為有人提到在以下論壇提到這裡討論這個問題,
http://groups.google.com/group/ade-scilab/browse_thread/thread/fb259973283c8ea2
所以我用 Scilab 寫了一個程序程序, 測出來的.
後來發現這裡列出了更多 ..
http://www.worldlingo.com/ma/enwiki/en/Prime_reciprocal_magic_square
—————–
這幾天研究了這種矩陣, 發現幾個規則 ：
若將真分數 1/P,2/P,….(P-1)/P 以 K 進位表示到小數點以下 (P-1) 位,小數點以下數字所形成的 （P-1)x(P-1) 矩陣有以下特性
1. 第 i 行及第 (P-i) 行, (1<= i <= (P-1)/2 )
為互補, 也就是和為 1
1 = .(K-1)(K-1)(K-1)….
例如十進位時
1 = .999999999…
九進位時
1 = .8888888….
2. 因此每列之和會毫不例外的為
(K-1)*(P-1)/2
, 所以若發現各列(豎)之和相等絕不稀奇, 因為
i/P + (P-i)/P = 1
3. 但各行（橫）相等也不算困難, 因為通常各行會形成抽象代數所說的循環群,一旦確定為循環群後，各行之和也必然相等但卻不一定和各列相等
甚麼條件下行,列之相等我就不知道了, 所以只能用 try 的
4. 要讓對角線和等於行, 列之和那又更少更困難了,
就像質數（素數）的產生沒有規則, 這種具幻方特性的矩陣可能沒有特殊公式來產生. 至多可以設計出有效率的檢定演算法來檢查一 P,K 整數組式是否可產生一幻方
哪位达人可以翻译下这个网页…… http://en.wikipedia.org/wiki/Prime_reciprocal_magic_square
太多数学名词了……
19, 383, 32327, 34061, 45341, 61967, 65699, 117541, 158771, 405817, 444287, 456503, 695389, 724781, 1102567, 1177859, 1498139, 2336989, 2695337, 3036857, 3249419, 3512749, 3571429, 4427299, 5141051, 7033823, 8324411, 9932179 这些都是的
我来说一个规律吧，刚要记忆的时候发现的。
比如1/19，从他的第18位小数1开始，乘以2，得到第17位小数2，依次类推可以得到所有的18位小数，但要注意有进位的；
一个原理，换个方向，从第1位小数也可以除以2，但第一位要看作10，依次去除，余数要当作10借给下一位，也可以得出整个18位小数。
借上面的方法，可以轻松记忆1/19的18位循环小数。
谢谢26楼提出的列表，试着将前面大于19的18个数依次除以19，得到如下结果：
383 / 19 = 20. 157894736842105263 157894736842
32327 / 19 = 1701. 421052631578947368 4210526316
34061 / 19 = 1792. 684210526315789473 6842105263
45341 / 19 = 2386. 368421052631578947 3684210526
61967 / 19 = 3261. 421052631578947368 4210526316
65699 / 19 = 3457. 842105263157894736 8421052632
117541 / 19 = 6186. 368421052631578947 3684210526
158771 / 19 = 8356. 368421052631578947 3684210526
405817 / 19 = 21358. 789473684210526315 789473684
444287 / 19 = 23383. 526315789473684210 526315789
456503 / 19 = 24026. 473684210526315789 473684211
695389 / 19 = 36599. 421052631578947368 421052632
724781 / 19 = 38146. 368421052631578947 368421053
1102567 / 19 = 58029. 842105263157894736 842105263
1177859 / 19 = 61992. 578947368421052631 578947368
1498139 / 19 = 78849. 421052631578947368 421052632
2336989 / 19 = 122999. 421052631578947368 42105263
2695337 / 19 = 141859. 842105263157894736 84210526
从结果中的小数后开始取前18位，组成一个新的18 X 18的矩阵：
1 5 7 8 9 4 7 3 6 8 4 2 1 0 5 2 6 3
4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6 8
6 8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3
3 6 8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7
4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6 8
8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6
3 6 8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7
3 6 8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7
7 8 9 4 7 3 6 8 4 2 1 0 5 2 6 3 1 5
5 2 6 3 1 5 7 8 9 4 7 3 6 8 4 2 1 0
4 7 3 6 8 4 2 1 0 5 2 6 3 1 5 7 8 9
4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6 8
3 6 8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7
8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6
5 7 8 9 4 7 3 6 8 4 2 1 0 5 2 6 3 1
4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6 8
4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6 8
8 4 2 1 0 5 2 6 3 1 5 7 8 9 4 7 3 6
很遗憾，期待的“幻方”没有出现，列之和不等于81。
因为这里的循环小数是按18位循环的，如果按照这个方法做出一个36 X 36 的矩阵，是否能得到期待中的那个不严格的“幻方”呢？
哪位大侠挑战一下？！
但是这个新的18 x 18 的矩阵还是多少保留了些许原本那个18 X 18矩阵的特点
1. 行之和是81。
2. 所有行都可以通过将第一行循环移动数位后得到。
发现这个规律的人，真有才@
7也是吧
好神奇咯 不错
按30楼的方法
循环节 分数 倍数
11111111 1/9 1
052631578947368421 1/19 2
0344827586206896551724137931 1/29 3
025641 1/39 4
020408163265306122448979591836734693877551 1/49 5
…..
写一个6*6的矩阵也满足这个性质，因为7的循环节长度是6
那么一个数字n的循环节长度为n-1，什么数才满足这个条件呢。
When someone writes an paragraph he/she
maintains the image of a user in his/her mind that how a user can understand it.
Therefore that’s why this post is outstdanding. Thanks!
For most recent news you have to go to see the web and on web
I found this web site as a finest site for most up-to-date updates.
Usually I don’t learn post on blogs, however I wish to
say that this write-up very forced me to take
a look at and do so! Your writing taste has been surprised me.
Thank you, very nice article.
Appreciating the hard work you put into your site and in depth information you present.
It’s nice to come across a blog every once in a while that isn’t the same unwanted rehashed information. Fantastic read!
I’ve bookmarked your site and I’m adding your RSS feeds to my
Google account.
Great post. I am facing a few of these issues as well..
I do not know whether it’s just me or if perhaps everybody else encountering
issues with your site. It appears as if some of the text within your content are running off the screen. Can someone else please
comment and let me know if this is happening to them as well?
This may be a issue with my internet browser because I’ve had this happen before.
Thank you
You really make it seem so easy along with your presentation but I to
find this topic to be really something which I think
I might never understand. It kind of feels too complex and very huge for me.
I’m having a look forward to your subsequent submit, I’ll attempt to get the hang
of it!
Howdy! Someone in my Myspace group shared this site with us so I came to look it over.
I’m definitely loving the information. I’m book-marking and will
be tweeting this to my followers! Wonderful blog and
amazing style and design.
Hello there! This post couldn’t be written any better! Reading this post reminds me of my
old room mate! He always kept chatting about this.
I will forward this article to him. Fairly certain he will have a good read.
Thanks for sharing!
Every weekend i used to go to see this website, because i want enjoyment, as this this web page
conations actually fastidious funny material too.
I think this is among the most vital information for me.
And i’m glad reading your article. But should remark on few general things, The site style is
great, the articles is really nice : D. Good job,
cheers
Hmm it appears like your site ate my first comment (it was super long) so I guess I’ll just sum it
up what I submitted and say, I’m thoroughly enjoying your blog.
I as well am an aspiring blog writer but I’m still new to the whole thing.
Do you have any suggestions for beginner blog writers? I’d genuinely appreciate it.
Fantastic beat ! I would like to apprentice even as
you amend your website, how could i subscribe for a weblog web site?
The account helped me a appropriate deal. I were a little
bit acquainted of this your broadcast offered shiny transparent concept
I do not even know how I ended up here, but I thought this post was good.
I do not know who you are but certainly you are going to a famous blogger if you aren’t already ;) Cheers!
I have read so many content about the blogger lovers except this post
is truly a fastidious piece of writing, keep it up.
First off I would like to say superb blog! I had a quick question in which I’d
like to ask if you don’t mind. I was curious to find out how you center yourself and
clear your head before writing. I’ve had a hard time clearing my mind in getting my thoughts out there.
I do take pleasure in writing but it just seems
like the first 10 to 15 minutes are usually wasted simply just trying to figure out
how to begin. Any ideas or hints? Many thanks!